Switched RL Series Circuit#
Consider the \(RL\) circuit shown below.
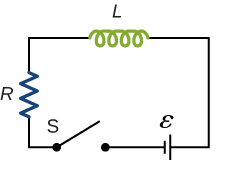
Here, \({\boldsymbol \varepsilon} = {{ params.emf }}\rm\ V\), \(L = {{ params.L }}\rm\ mH\), and \(R = {{ params.R }}\rm\ \Omega\).
Part 1#
Determine the time constant of the circuit.
Answer Section#
Please enter in a numeric value in \(\rm\ s\).
Part 2#
Immediately after switch \(\rm S\) is closed, what is the initial current through the resistor?
Answer Section#
Please enter in a numeric value in \(\rm\ A\).
Part 3#
What is the final current through the resistor?
Answer Section#
Please enter in a numeric value in \(\rm\ A\).
Part 4#
What is the current through the resistor when \(t = t^{\star} = {{ params.num }} \tau_L\)?
Answer Section#
Please enter in a numeric value in \(\rm\ A\).
Part 5#
What is the voltage across the inductor when \(t = t^{\star} = {{ params.num }} \tau_L\)?
Answer Section#
Please enter in a numeric value in \(\rm\ V\).
Part 6#
What is the voltage across the resistor when \(t = t^{\star} = {{ params.num }} \tau_L\)?
Answer Section#
Please enter in a numeric value in \(\rm\ V\).
Attribution#
Problem is from the OpenStax University Physics Volume 2 textbook, licensed under the CC-BY 4.0 license.
