Rolling Disk of Spruce and Steel#
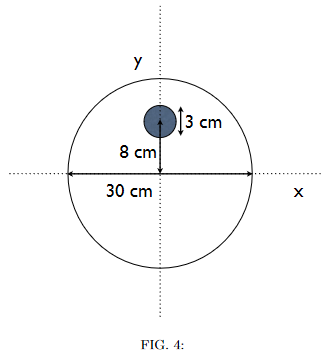
A disk that will appear to roll uphill is made from spruce (\(\rho\_{\text{spruce}} = \) 462.0 \(kg/m^3\)) and steel (\(\rho\_{\text{steel}} = \) 7830.0 \(kg/m^3\)) by drilling a 3 \(cm\) diameter hole, centred 8 \(cm\) above the centre of a 30 \(cm\) diameter spruce cylinder, as shown in the figure. Into this hole is inserted a piece of steel of identical dimensions. The disk has a thickness of 10.3 \(cm\).
Part 1#
Calculate the mass of the spruce removed from this disk.
Answer Section#
Please enter in a numeric value in \(kg\).
Part 2#
Calculate the mass of the steel that replaces the spruce.
Answer Section#
Please enter in a numeric value in \(kg\).
Part 3#
The location of the centre of mass of the weighted disk is closest to:
Answer Section#
\(x\) = 1 \(cm\), \(y\) = 0 \(cm\)
\(x\) = 0 \(cm\), \(y\) = 1.0 \(cm\)
\(x\) = 0 \(cm\), \(y\) = 2.0 \(cm\)
\(x\) = 0 \(cm\), \(y\) = 3.0 \(cm\)
\(x\) = 0 \(cm\), \(y\) = 4.0 \(cm\)
\(x\) = 0 \(cm\), \(y\) = 0.0 \(cm\)
Attribution#
Problem is licensed under the CC-BY-NC-SA 4.0 license.

