Monkeys#

(https://makeagif.com/gif/gibbon-brachiation-a5VJFW) sourced from this original YouTube video (https://www.youtube.com/watch?v=acy–k7Qww0)
Brachiation is a form of locomotion (movement) where primates swing from tree limb to tree limb using only their arms. During brachiation, the body is alternately supported under each forelimb. Source: https://en.wikipedia.org/wiki/Brachiation
Part 1#
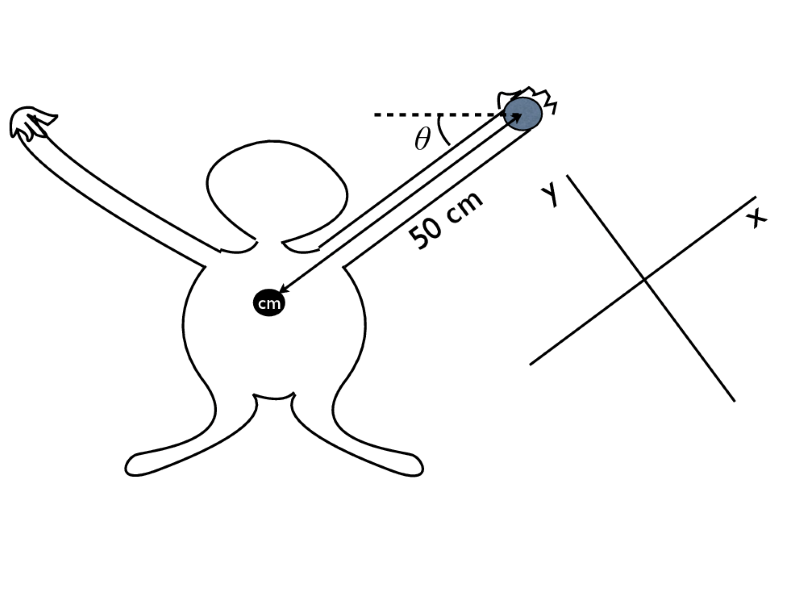
In brachiation, monkeys move from tree branch to tree branch by swinging from their arms. In the position shown in the figure (left), draw a free body diagram on the provided axes in the figure (right) for the monkey, assuming that it is momentarily at rest. (Label the forces \(\vec{F}\) and \(\vec{W}\) with subscripts on each, and include the angle).
Let the mass of the monkey be 10 kg, \(\theta\) = 30 \(^{\circ}\) , and the centre of mass of the monkey to be 50 cm from its centre of mass as shown in the figure.
Answer Section#
File upload box will be shown here.
Part 2#
Calculate the torque exerted by \(\vec{F\_{m\ on\ b}}\) about the branch.
Answer Section#
Please enter in a numeric value in m.
Part 3#
Calculate the torque exerted by the mass of the monkey about the branch.
Answer Section#
Please enter in a numeric value in m.
Part 4#
The moment of inertia of a solid sphere about its centre is \(\frac{2}{5}\) \(M\)\(R^{2}\) . If the the mass of the monkey can be treated as a sphere of radius 30 cm. Find the moment of inertia of the monkey about its centre.
Answer Section#
Please enter in a numeric value in m.
Part 5#
The parallel axis theorem states that moment of inertia of an object of mass \(m\) about an axis, a distance \(d\) from its centre of mass is given by \(I\) = \(I\_{cm}\) + \(m\)\(d^{2}\) . Find the moment of inertia of the spherical monkey about the branch.
Answer Section#
Please enter in a numeric value in m.
Part 6#
Find the angular acceleration of the monkey.
Answer Section#
Please enter in a numeric value in m.
Part 7#
Find the tangential acceleration of the centre of mass of the monkey about the branch.
Answer Section#
Please enter in a numeric value in m.
Part 8#
Your result from part 7 is not the same as you would get from solving for the acceleration from the free body diagram in part 1. Explain why this is, referring to the particle model.
Answer Section#
Answer in 2-3 sentences, try and use full sentences.
Attribution#
Problem is licensed under the CC-BY-NC-SA 4.0 license.

