Turtle on a log

Turtle on a log#

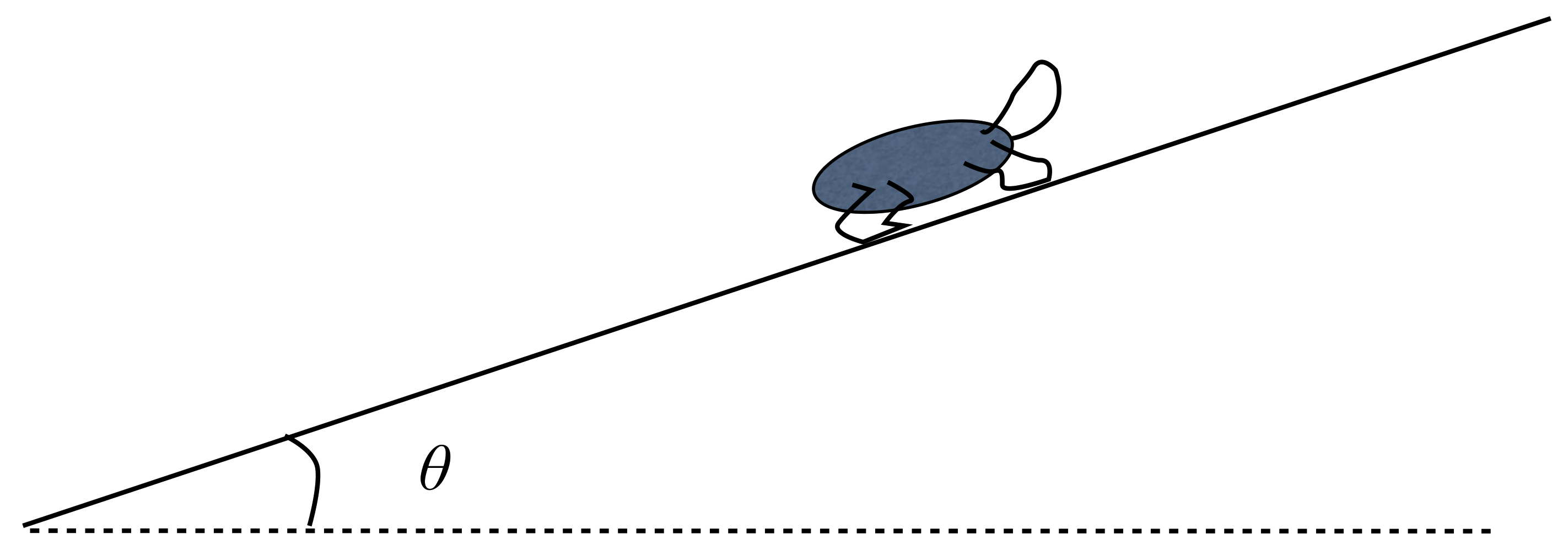
A turtle lies on a log in the sun as shown in the figure below. The turtle has mass \(\rm m\), the log makes an angle \(\theta\) with respect to the horizontal and the coefficient of static friction between the turtle and the log is \(\mu_s\) (where \(\mu_s > \tan\theta\)).

Part 1#

The magnitude of the normal force, \(F_n\) is:

../../../../../_images/turtleonlog.png

Answer Section#

  • \(F_n = mg\)

  • \(F_n = 0\)

  • \(F_n = mg \sin\theta\)

  • \(F_n = mg\cos\theta\)

  • \(F_n = 2\cdot mg tan\theta\)

Part 2#

The magnitude of the static frictional force \(F\_{fs}\) is:

Answer Section#

  • \(F_{fs} = \mu_s mg\)

  • \(F_{fs} = \mu_s mg \cos\theta\)

  • \(F_{fs} = \mu_s mg\sin\theta\)

  • \(F_{fs} = mg\sin\theta\)

  • \(F_{fs} = mg\cos\theta\)

  • \(F_{fs} = mg tan\theta\)

  • \(F_{fs} = \mu_s mg tan\theta\)

Attribution#

Problem is licensed under the CC-BY-NC-SA 4.0 license.
The Creative Commons 4.0 license requiring attribution-BY, non-commercial-NC, and share-alike-SA license.