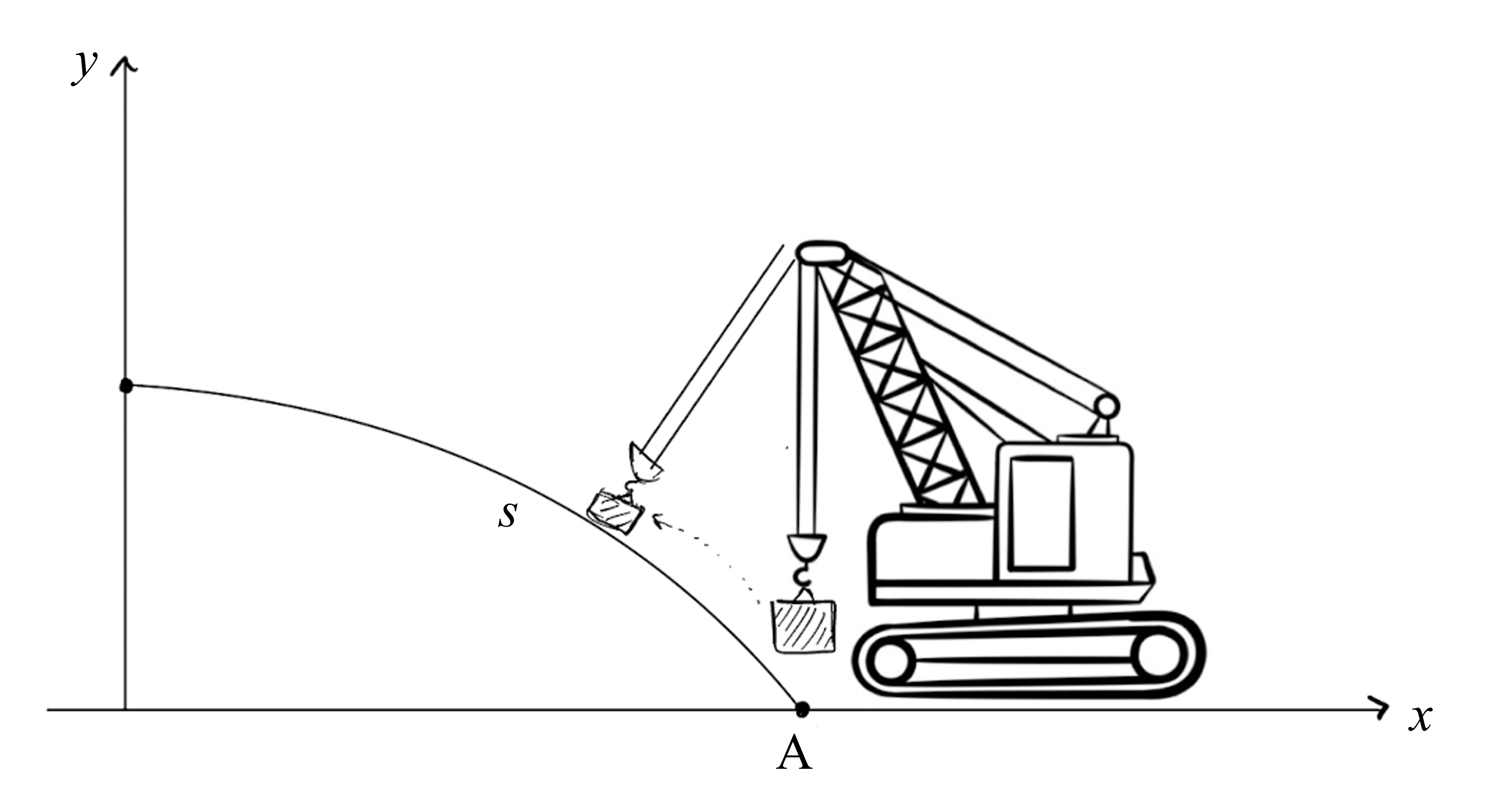
Curvilinear Motion of a crate carried by a crane#
A crane moves a crate through point A with a speed of \({{ params.v_0 }}\ \rm{m/s}\) and increases the speed constantly at a rate of \({{ params.a }}\ \rm{m/s^{2}}\).
Find the magnitude of the crate’s acceleration when the arc length is \({{ params.s }}\ \rm{m}\) and when \(x_0 =0 \ \rm{m}\).
The equation of the arc is \(y= m - \frac{x^{2}}{w}\)
\(w = {{ params.w }}\ \rm{m}\), \(m = {{ params.m }}\ \rm{m}\)
Part 1#
Find the speed \(v_2\) when \(x = 0\ \rm{m}\).
Answer Section#
Please enter the speed in \(m/s\).
Part 2#
Find the radius of curvature.
Answer Section#
Please enter the radius in \(m\).
Part 3#
Find \(a_n\) at the same point.
Answer Section#
Please enter the acceleration in \(m/s^{2}\).
Part 4#
Find the total acceleration.
Answer Section#
Please enter the acceleration in \(m/s^{2}\).
Attribution#
Problem is licensed under the CC-BY-NC-SA 4.0 license.

